The mathematics classroom environments of the past consisted of textbooks, worksheets, and rows of desks. This model catered to the rote learning model that killed student creativity and neglected the students’ ability to problem solve using their own methods and strategies. As an educator entering the teaching profession in the 21st century, I hope to bring a wave of fresh ideas and new approaches to learning that engage students, challenge their thinking, and promotes a positive growth mindset.
Role of the Educator
As an educator, I embrace the opportunity to create a learning environment in which students are able to flourish as learners and as people, developing into positive and active members of society. I think of myself as a ‘facilitator of learning,’ where I provide authentic learning opportunities for my students to inquire and discover as I continue to learn alongside them. By providing opportunities for guided experiential learning, rather than structured rote teaching, students learn by doing, writing, designing, creating, making and solving, not just by listening.
Within the classroom, the educator should circulate around the learning environment, checking in with each student or group of students to assess their learning, write anecdotal notes, and challenge or extend student learning. They can also identify which students require more academic assistance, especially those with math IEPs, and work with the students to find activities that develop specific learning goals.
More than anything, it is the role of the educator to build strong, positive attitudes towards math. As outlined in the Ministry of Education’s Parent Guide to Doing Mathematics with your Child, “When children feel positively engaged and successful, they are more likely to stick with an activity or a problem to find a solution” (pg. 2). A positive growth mindset will help students to exceed their own expectations!

Manipulatives
Manipulative allow students to engage in experiential learning opportunities in which they can be hands-on and physically experience the mathematical learning. They should be readily available to students to use during any math task, thus providing a consistent and hands-on aid to their learning. Students should have access to a ruler and calculator for everyday use, both of which can easily be stored in the students’ desks or supply bins. Here are some examples of manipulatives that could be available in containers or shelving units within the classroom:
- Base Ten Blocks: great for showing differences in quantities, helping students when counting, visually showing multiplication, and reinforcing proportional reasoning
- Centimeter Cubes: used for counting, creating bar graphs, and measuring perimeter, area, and volume
- Tangrams: reinforce themes within geometry, such as shapes, sides, angles, and vertices
- Measuring Cups: used to practice measurement and comparing volumes and quantities of these measurements
- Tape Measures: valuable practice measuring tangible items within the classroom environment while using different metric systems (i.e. mm, cm, dm, m, etc.)
- Thermometers: applicable real-life measurement, students can measure the temperature of various items (outside temperature, classroom fish tank, bowl of snow, etc.) and track measurement overtime
- Money: great for teaching financial literacy, reinforcing adding and subtracting, and promoting play-based learning
- Fraction Strips: assists students with proportional reasoning, provides a visual representation of various fractions, and students can stack them on top of each other to view differences in quantity and size
- Pattern Blocks: promotes geometric concepts and allows for creativity (i.e., building shapes using smaller shapes)
- Protractor: used to measure angles of objects, assists students in developing geometric comprehension
- Graph paper: can be used for graphing data, measuring perimeter and area, and designing floor plans

Mathematics Technology
The use of technology within the mathematics classroom can help to engage students in their learning, while also providing opportunities to work with different aids throughout their problem solving. Here are a number of ways in which technology can be integrated throughout the math classroom.
Kahoot is an online tool that engages students in a class-wide quiz about a certain topic. The quiz can be on any topic, including multiple concept areas in mathematics. Students read a question, choose the correct multiple choice answer and their results are recorded. Even with so much learning taking place, Kahoots always seem to bring out the fun and excitement in the lesson. The students are happy because they get to use technology and they also feel a sense of competition, which in my opinion furthers their overall performance.
Another digital tool that can be used within a math classroom is Quizizz. This program is very much like Kahoot, but students work through the quiz independently, at their own pace, and the order of the questions get randomized so students don’t shout out all of the answers. It still ranks the students based on the number of answers correct and generates reports for the teacher to review. Quizizz is a great tool!

Osmo has a number of different kits that you can buy. As long as the school has an iPad and can download the corresponding app, all that will need to be purchased are the Omso kits. These kits come with the iPad stand, the curved mirror for the camera, and the tangram pieces. They also have other kits about numbers, coding strings, and word tiles.

There are a number of online math games that students can easily access to help promote concepts being taught in class. An example of a game that students can access is Prodigy. This game allows students to create a profile, design an avatar, and complete multiple levels. Students have to complete math questions to battle other characters and progress through the levels. More times than not, students will be so consumed with the game that they won’t even realize how much math learning is taking place!

Coding is a great cross-curricular connection between Math, Science, and even Language. Coding has students create sequences of commands that lead to a specific action or outcome. When using robotics technology, such as a Sphero, students are able to code the robot to move a certain distance, rotate a specific way, and even travel at a certain speed. Robotics and coding would typically fall under the category of Science and Technology, however, there are many different and creative ways in which it can have a math focus. For example, students could use angles and rotations to maneuver the robot through a maze that the students create. There are also some valuable Language expectations met when coding, predominantly procedural writing. Even without robotic technology, students can practice coding online at Code.org, a free website that providing coding tutorials and challenges for students. Coding and robotics are great ways to bring a math problem to life, while also teaching the students valuable and applicable 21st century skills.
Khan Academy is an online learning platform on which students complete different modules and assess themselves by completing different tasks. The students’ profiles are linked to the teacher’s account, allowing the teacher to see how much time has been spent on Khan Academy and how much progress they have had in each module. The various tasks and instructional videos provide students with supplemental learning that can be accessed both within and outside of the classroom. Once or twice a week, students could come into the classroom with a Chromebook on their desk and a new module on Khan Academy assigned to them. From what I was told, this resource is based out of the USA, so there are some issues with measurements (gallons vs. litres), but for the most part, the curriculum standards and expectations are concurrent with Ontario’s curriculum.

Learning Centres
Learning centres allow students the freedom of choice when it comes to their learning. These centres are readily equipped for students to go to and begin a task. They also provide many inquiry-based learning opportunities, especially since the centres are student-driven.
As outlined in the previous section, technology provides many great learning opportunities that may not otherwise be available. Computers, iPads, robotics, and other applications truly add to the learning environment and help students to gain imperative 21st century technology skills. Having technology learning centres available to students provides continual exposure to these important concepts.
In addition to the technology centres within the classroom, there should be some sensory stations that allow students to be hands-on with their learning. For primary grades, water and/or sand tables provide students with an environment in which they can play and explore, while using multiple math concepts, such as measurement. In the Junior grades, students could use scrap pieces of wood and various tools to practice applicable building and woodworking skills. These activities would also promote math learning through geometry, measurement, and number sense.


Games help to encourage play-based learning and show students that math does not have to be dry and scary. A variety of math games could be available at a learning centre in the classroom. Possible games could include playing cards with a binder of multiple card games (each game is strategy chosen to reinforce a mathematical concept), dice for probability games or operation races (i.e., first to multiply the numbers of the rolled dice together), and flashcards to quiz each other and race to be the first to answer the question correctly.

Classroom Layout
The classroom should be organized in a way in which each area is used effectively and is organized. Bins and shelving units are a great way to store manipulatives and electronics so that they are safe and easily accessible for the next person. The different areas of the room should be labelled so that students know what is available to them and where they can find things within the classroom.
There should be a variety of learning areas in which the students can choose to work at, such as:
- Classroom carpet: centred around a SMART Board or whiteboard for introducing a new topic, consolidating a lesson, having math discussions, doing number talks, or other group activities, such as Which One Doesn’t Belong
- Student-teacher conference area: area assigned for check-ins and working with specific students (such as students struggling with a concept or needing extra assistance)
- Tables: promotes collaboration, teamwork, and communication
- Cushions on floor: can be used for both individual learning and group work
- Standing tables: considers embodied learning by providing an area for students to stand while working
- Study carrels: an individualized quiet area that limits distractions and provides a safe space for students
The classroom will have evidence of student learning up on the walls for students to reference. This gives a sense of ownership and agency to the students knowing that their work is being displayed. It also allows students to reflect back on what they have learned and refresh themselves about strategies to complete a given task. In addition to student work, a word wall can be used to reinforce conceptual language and knowledge.
Success criteria are an important and successful way of communicating the academic expectations for a given task to students. This concept has developed by engaging students in the process of defining the learning goals and outlining what success looks like. This idea of co-creating success criteria is a great way to encourage students to use assessment vocabulary while also making them more aware of identifying what is expected of them given any assessment description. It also encourages the concept of students taking ownership of their own learning and deciding what the focus of assessment should be on. The success criteria should be explicitly labeled and posted in the classroom for students to see.

Inquiry-Based Learning
Problem solving questions, specifically in math, provide opportunities for students to practice their learned skills in applicable and relevant situations. They challenge the students to reflect on what they have learned theoretically and apply this knowledge in practical, thought-provoking applications. It is very important that we teach students to embrace problem solving, treating it like a puzzle to be solved rather than a brick wall preventing us from achieving success. When we adequately prepare students with the tools that they need during problem solving, they come to learn that they are able to problem solve and they can achieve success. This, in turn, develops a positive disposition towards problem solving for our students.
The Guide to Effective Instruction: Grades K to 6 – Volume 2 – Problem Solving and Communication teaches us that rich and engaging problem solving questions not only teach students through problem solving (practicing conceptual understanding), but they also teach student about problem solving by learning applicable learning skills (Guide to Effective Instruction, pg. 6). By teaching student through and about problem solving, we are able to see if the student has grasped the concept while also exploring the strategy they used throughout the process. When we are able to see both aspects, we then know that we have created a rich mathematical question. It is also important that we ensure the questions are relevant to the students by using real-world situations that are linked to their specific interests.

Conversations around problem solving help to teach students to be cognitive about their own strategies, while also being able to learn from their peers and adopt new and perhaps more efficient strategies. As Marion Small says in the video Open Questions and Contexts, “[Different strategies] enrich the conversation; it does not detract from it.” Math talks and bansho consolidation presentations are great ways to verbally explore these strategies in a whole-class setting. Other ways to communicate their thinking could be in a math journal, in which the student explains the strategies they used throughout the day’s lesson, or by creating a video/voice recording of their verbal explanations (for those students less inclined to share with the class).
As Doug Clements said in his talk about Intentional Play-Based Learning, the best type of learning including all kinds of learning experiences, including both play-based and guided learning. We should prompt students and give creative challenges that develop high-caliber mathematical thinking and reasoning while the students are engaging in play-based learning. This model of inquiry-based learning also provides the teacher with multiple opportunities to check-in with students throughout the learning, help to reinforce mathematical language, and develop the student’s ability to explain their processes and strategies. Students develop higher levels of social skills, emotional skills, and self-regulation skills when they emerge in guided play-based learning.

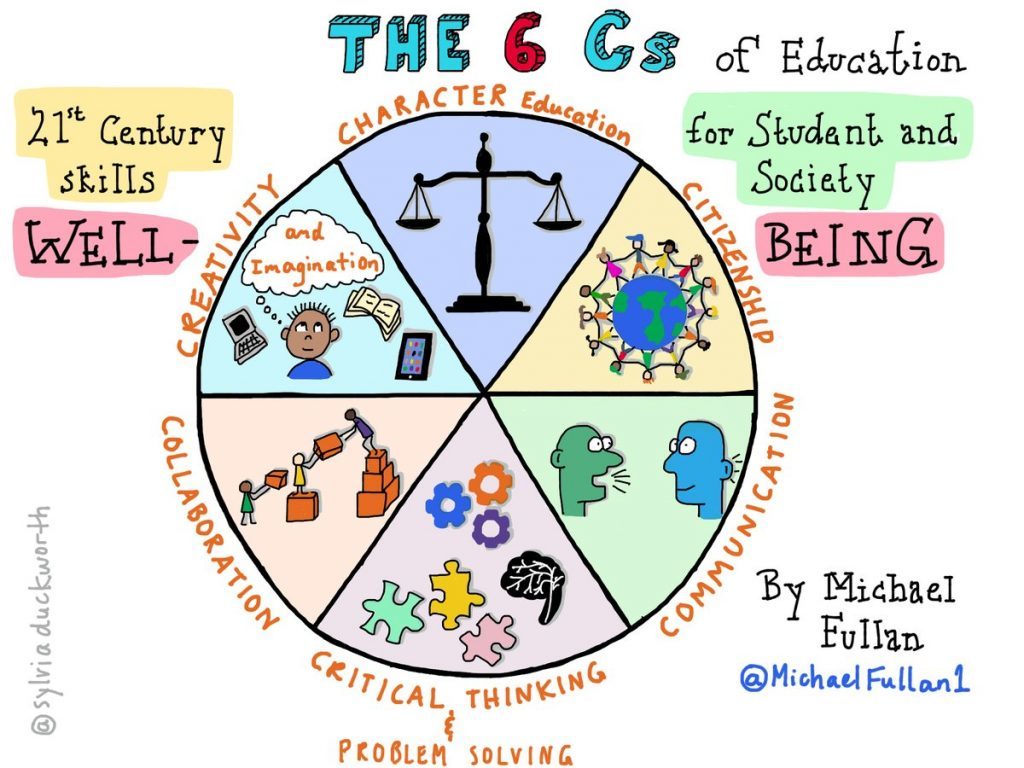
My goal when providing students with inquiry-based learning opportunities is to achieve multiple curriculum expectations, while also working on many learning skills. The 6 C’s are a great framework when considering the learning skills that students could work towards in a given task. The 6 C’s outline some important 21st century learning skills that each student should work towards developing.